Written By: Jaclyn Mazzone, Fifth Grade Special Education Teacher at P.S. 94 in Brooklyn, NY

Working in special education, I help students with special needs as well as other students who struggle with math. One of the most beneficial features of Exemplars is the ability to differentiate easily for struggling learners. Some need just a little extra support through small group instruction. The more accessible version of Exemplars tasks is perfect for them!

The more accessible versions present the same problem-solving elements as the grade-level task and the more challenging task, but in a simplified form: they’re less wordy, involve simpler numbers, and require fewer steps to complete. At the same time, they’re still demanding enough that they challenge each student to think more deeply to determine what the question is asking, decide what information they need to know, choose a strategy to solve the problem, complete the mathematical computations, and show their work.

Repetition Is Key

Specifically in my class, I’ve noticed repetition is key. My students complete Exemplars at least once a week, so they’ve been able to build a routine. A colored version of the Problem Solving Process is provided for each student. It has become automatic for them to carry out the first two steps of the Procedure independently. They start out by reading the problem twice; the first time they just read it, and the second time they annotate, highlight/locate important information in the problem, and underline the question. Once they’ve determined what the problem is asking them to do, they then move on to writing their “I have …” and “I will …” statements independently. Next, as a group we read the entire problem together — their third time to read it — ensuring that the important information has been located, underlined, or highlighted, and that they understand what the problem is asking them to figure out. Many of my students are English Language Learners so language is also sometimes an issue for them. Therefore, we carefully break apart the math tasks sentence by sentence. We address any unknown vocabulary and I encourage them to use their prior knowledge to connect to the problem.

Small-Group Discussion and Scaffolding

Then, the students engage in a small-group discussion and discuss what the problem is about. They brainstorm different strategies that could be used to solve the problem and discuss why those strategies would work. Then I step in to scaffold and guide them to set up their chosen strategy correctly, whether it be a table, number line, diagram etc. Once I’ve supported them in setting up their chosen strategy and answered any questions they might have, they work with their small group to continue solving the problem. The students know to show all of their mathematical thinking on paper — this expectation has been instilled since day one of their work with Exemplars. As they work, I circulate around the room to provide one-on-one support where necessary.

After I allow approximately 20-25 minutes for them to complete the problem with their peers, we come back together as a group and discuss the different ways we solved it. The students then move on to the next step of the Problem Solving Procedure by making mathematical connections. I encourage them to try to extend the problem, write what they notice in their work, or try to find a pattern. Because connections are still rather challenging for them, I link the task to our current unit of study or math topics we have previously worked with to help them make those connections.

The Importance of Tools

Tools are important resource for my students. Working with learners in a small group, I am able to accommodate their different learning styles. Depending on the task, I use manipulatives such as connecting cubes, fraction bars, unit cubes, or sometimes even a basic multiplication table chart to further support their needs.

Peer Assessment

My students also peer-assess each other’s work. They swap papers with a partner and use the Exemplars student-friendly rubric to score their partner’s work. Since the student-friendly rubric addresses the same criteria as the teacher rubric in a simpler form, this practice has made them very familiar with the four levels of achievement and what’s required to become a Practitioner (meet the standard).

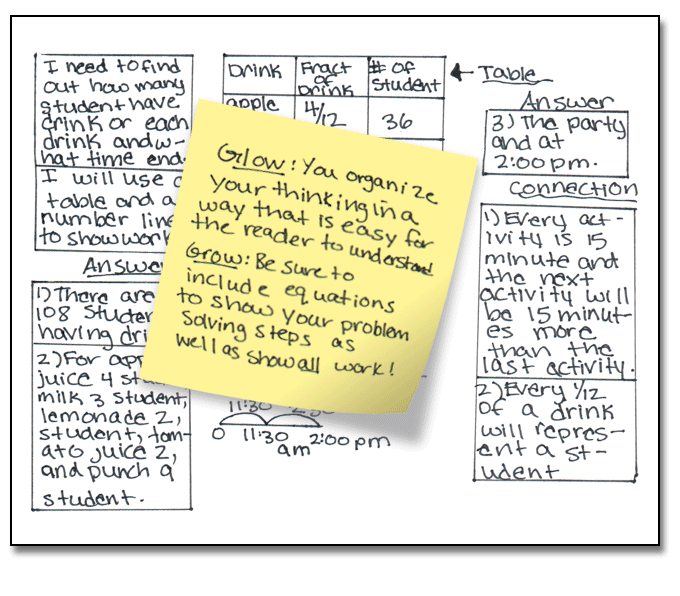
Peer assessment has also built up their self-confidence. As they assess each other’s work, I ask them to find a “Glow” statement — something their classmate did well — and a “Grow” statement — an area for improvement. In doing this, they notice that other students have challenges, too, and might even make the same mistakes as they do. Peer assessment has not always been an easy task for them, but through repetition and teacher feedback, the students have picked up on sophisticated math language to use when providing helpful Glow/Grow comments to peers.

An example of a student’s peer-assessment comments using the “Glow and Grow” strategy. 

Exemplars has been a very beneficial tool in my classroom to reinforce the mathematical concepts we learn throughout the year. Through careful scaffolding and support, my students have learned to persevere when problem solving, show all of their mathematical thinking, use different strategies to achieve the same answer, and provide peer feedback. My students consistently aim to achieve a level of Practitioner and some even aim for Expert. Exemplars has become a weekly activity in my classroom. The students truly find it enjoyable!

Jaclyn Mazzone’s Biography

Jaclyn Mazzone is a fifth-grade special education teacher at P.S. 94 in Sunset Park, Brooklyn. She has a master’s degree in special education from Touro College and a bachelor’s degree in childhood education from the College of Staten Island.